Derivation of Clausius Inequality Formula

This is a consequence of the second law of thermodynamics, which is true for all possible cycles, including (reversible, irreversible, heat engines, and refrigerators). 

We can represent any reversible cycle by a series of Carnot cycles. So we can consider a Carnot engine from which Clausius's inequality is derived.

{tocify} $title={Table of Contents}

Clausius Inequality Derivation

Let the cycle of a reversible engine work between two temperatures T1 and T2. The cyclic integration of heat transfer for these cycles Q1 and Q2 is greater than zero.

Q ⸹Q = Q1 - Q2 ≥ 0

T1 and T2 is constant

Փ⸹Q/T = Q1/T1 - Q/T2

If by placing T1 ⟶T2 Փ⸹Q = 0

Then 

Hence for the precycle of the reversible engine

Փ⸹Q = 0, and Փ⸹Q/T = 0

Now consider an irreversible heat. The same temperature works between T1 and T2. And the same amount of heat is received by Q1 and given off by Q2. Comparing an irreversible engine with a reversible engine, the second law of thermodynamics states that

Wirr >Wr.

Now for the reversible cycle engine

Փ⸹Q = Q1 - Q2 ≥ 0

And

Փ⸹Q/T = Q1/T1 - Q/T2 < 0

Making the cycle more irreversible Π⸹Q = 0 and the value of Π⸹Q/T becomes negative. Thus for all irreversible heat engine cycles٠

Փ⸹Q ≥ 0 & Փ⸹Q/T < 0

Similarly, we can show reversible and irreversible cycles.

Փ⸹Q ≥ 0 & Փ⸹Q/T = 0

Փ⸹Q <0 & Փ⸹Q/T < 0

In this way, we can show all the cycles.

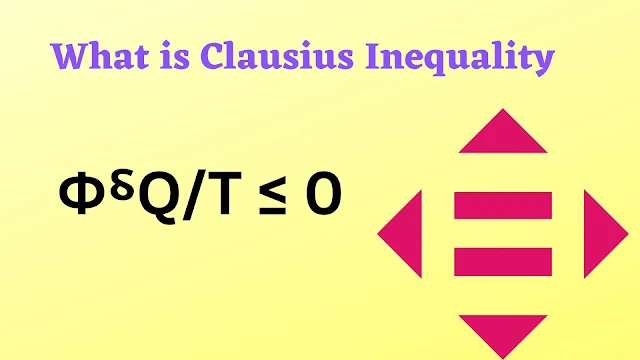
Clausius inequality formula 

Փ⸹Q/T ≤ 0

The above equation is called inequality Clausius.

Also Read: 

Second Law of Thermodynamics in Terms of Entropy

When all the Entropy Changes in a Process are Included

What is Clausius's Inequality?

Definition of Thermodynamics Equilibrium 

When there is no unbalanced force between a system and its surroundings, the system is said to be in mechanical equilibrium. If there is no tendency within the system to change its internal structure ie chemical reaction and parallelization of matter from one part of the system to another, then the system is said to be in chemical equilibrium.

If all the parts of the system are at the same temperature which is equal to the temperature of its surroundings, the system is said to be in thermodynamic equilibrium. In this case, there is no change in the state of the system or its surroundings.